In bachelor lectures of thermodynamics, systems are mostly considered to be homogeneous. However, spatially inhomogeneous features are omnipresent in everyday life.

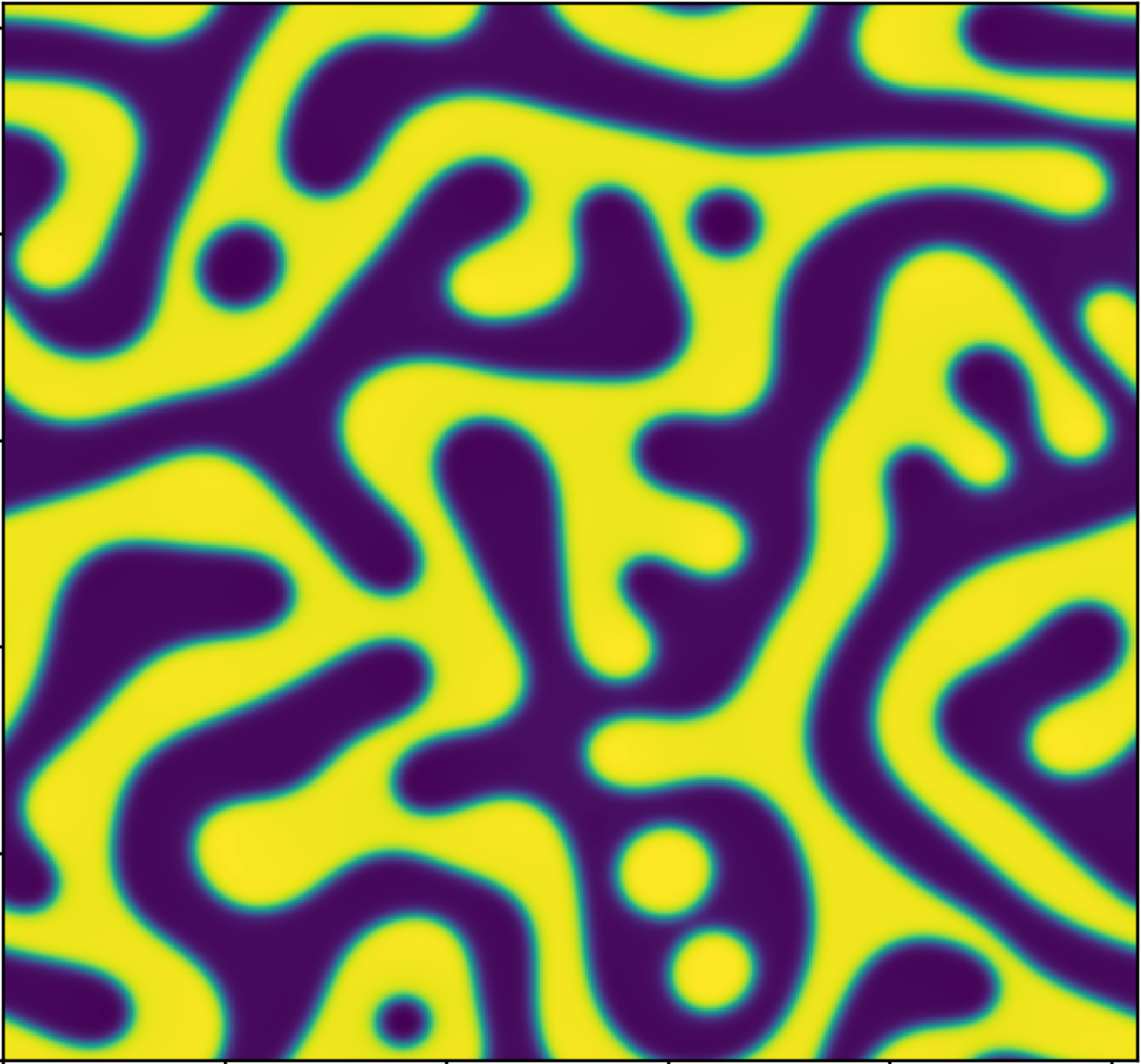
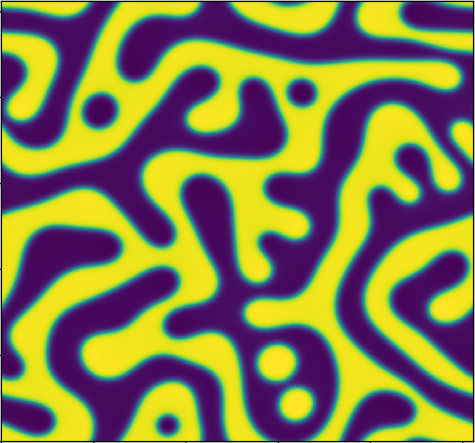
These range from non-equilibrium phenomena like pattern formation which can occur as the consequence of reaction-diffusion models to simple systems of two or more non-reacting chemicals that show phase separation. The latter can be easily reproduced by mixing oil and water causing a phase separation and dynamic coarsening of small droplets of oil in water until there are only two phases left.

In this work, we will focus on components that do not react with each other and the total constitution does not change over time.

For mixtures of two (or more) components that do not react with each other, the thermodynamics can be investigated with the Flory-Huggins theory, which can predict whether the system stays in a homogeneous form or phase separates into two phases. The Cahn-Hilliard equation, on the other hand, provides a continuous description of the phase separation dynamics of such multi-component mixtures.

It can also model other mechanisms in nonequilibrium pattern formation, such as the process of nucleation and growth, and the coarsening dynamics of clusters of the different phases.

We will investigate the phase separation of two-component systems described by the Cahn-Hilliard equation. Specifically, we will construct the phase diagram of the system from the Cahn-Hilliard model analytically with help of linear stability analysis and we will verify the results and investigate the dynamics of phase separation using numerical simulations qualitatively and quantitatively by studying properties such as the growth rate of clusters over time.